Friday 20 October 2017

define ratio , proportion , direct and inverse variation

Define ratio , proportion , direct and inverse variation

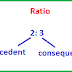
Ratio:

The relationship b/w two quantities are known as ration.
a:b  is written as a/b

Proportion:

The relationship b/w two ratios are known as proportion.
a:b=c:d is written as a/b=c/d etc.

Direct variation/proportion:

If we have two quantities and one quantity is going to increase then another quantity is also going to increase this type of variation is called direct variation.

Example:

  • ·         If the prize of 2 pens is 20 rupees then the prize of 4 pens are 20.In this statement the quantity of pens are increase and the cost of pens are also increase.

  • ·         If the prize of 6 books is 600 rupees then the prize of 2 books are 200 rupees. In this statement the quantity of book is increase that why the cost of book are also increase.

Inverse proportion/variation:

If we have two quantities and one quantity is going to increase then another quantity is  going to decrease then this type of variation is called inverse  variation.

Example:

  • ·         If 5 mans can complete a 5 m wall in 10 days. If we increase the quantity of mans then the day will decrease. Means that 10 men can complete the same work in 5 days. In this statement we increase the quantity of mans that’s why the quantity of day/time is decrease.

  •         If 20 mans can dig a hole in 8 days. If we increase days then the quantity of men’s will decrease. In 16 days only 10 men’s can do the same work .in this statement the quantity of day will decrease because of the quantity of men’s are increase.







Posted by Unknown on 03:47  No comments »

Saturday 14 October 2017

types of quadratic equation


Types of quadratic equation and their difference 

Quadratic equation:

An equation which having square of unknown (variable) quantity, but no higher power is known as quadratic equation. The standard form of quadratic equation in x variable is ax2+bx+c = o. whereas a is the coefficient of x2, b is the coefficient of x and c is the constant term.   a,b,c  all are real numbers and x is an known variable.
For example
Standard form example                                                           not Standard form example        
·         ax2+bx+c = o                                                                                  ax2+bx = -c
·         4x2+3x+1 = o                                                                                  4x2+3x= -1
·         7x2+2x+8 = o                                                                                  7x2+2x = -8
·         x2-6x-9= o                                                                                        x2-6x = 9
·         6x2-x+1= o                                                                                       6x2 +1 = x

Pure quadratic equation:

Pure quadratic equation is form of quadratic equation in which the term bx is absent.
It means in quadratic equation (ax2 + bx + c = 0), if b=0 then this equation is called pure quadratic equation. The form of this equation is ax2 + c = 0 whereas a,b are real numbers and x is known term called variable.
For example
·         ax2 + c = 0
·         5x2 + 6 = 0
·         px2 + q= 0
·         10x2 -10 = 0
·         2x2 - 18 = 0

Main difference b/w pure quadratic equation and quadratic equation.

The main difference b/w these two equations are the term bx.
·       It means if in any quadratic equation b=0 then this time it is known as pure quadratic equation but if b=0 then this time it is known as quadratic equation.
·         In pure quadratic equation the term bx is absent.
·         In pure quadratic equation the term bx is must be present.

Methods to solve quadratic equation.

v  Factorization method
v  Completing square method
v  Quadratic formula

Methods to solve pure quadratic equation.

v    factorization method 

pure quadratic equation may be solved by using the property of square roots of equal numbers.




Posted by Unknown on 01:36  No comments »

Thursday 12 October 2017

what is perimeter and area

what is perimeter and area

Area:


Area is defined as the indicated number of square unites needed to fill a region on a flat surface or simple figure.  For rectangle, the area is computed by multiplying its length and width. The rectangle has length of 4 units and width of 8 units, then the area of this rectangle is equal to 32 square units. It means that 32 squares are required to fill this region. 



Perimeter:

Perimeter is defined as the whole distance around the exterior on a flat surface. It is the total length of the boundary that enclosed the interior region. 





Let solve different examples which help to understand area and perimeter.

1 1-      A square having 7 m side. Find the area of the square.

We know that the sides of a square is equal in length that why,
Length of square = width of square,
So the area of square = length x length or side x side
Area of square = 7m x 7m = 49 m2

2 2-       The length of the side of pentagon is 50 cm find its perimeter in meter.

First of all we convert cm into meter
We know that 1 meter is equal to 100 cm
So,
50 cm= 50/100m=0.5m

Let’s find the perimeter of pentagon.
 We know that pentagon has 5 sides and all sides are equal in length.
Suppose the side of pentagon is x then,
                                                                                                                          Value of side= x = 0.5 m
 Perimeter = x+x+x+x+x                                                   
Perimeter=0.5m + 0.5m + 0.5m + 0.5m + 0.5m = 2.5m.

3 3-      The area of a square field is 121 m2. Find the length of a side of a square.

Suppose the side of square be y
We know that area of square= side x side=y x y 
Area = y2
                                                                                     Put the value
121m2 =y2                                       

√121  =  √y2                                                Now root on both side

11 = y

The length of the side of square is 11 m


For father details
https://www.khanacademy.org/math/geometry/hs-geo-foundations/hs-geo-area/v/perimeter-and-area-basics                   
Posted by Unknown on 23:31  No comments »

Discriminate 

Discriminate means to make difference.but in mathematics we use discriminate formula to find the nature of the roots of quadratic equation without solving the quadratic equation.

Discriminate formula

D   =   b2 - 4ac

where ,

D = Discriminate

a,b and  are the coefficients

c is content term of quadratic equation   

Rules for nature of root of quadratic equation 

If discriminate is zero (D = O) then the roots of this equation are real and equal.

If discriminate is in negative (D = - answer) then roots of this equation are unequal and imaginary. 

If discriminate is positive and perfect square then roots of this equation are real,unequal and rational. 

If discriminate is positive but not perfect square then roots of this equation are real,unequal and irrational. 

examples 

1-     4x2+ 3x+2=0

solution
a=4 , b=3 , c=2
 
D   =   b2 - 4ac  put the values in formula
      
D   =   (3)2 - 4(4)(2)
D   =   9-32
D   =   -23
D   =   negative so root of this equation are unequal and imaginary.


2-    3x2+ 5x-1=0

solution
a=3 , b=5 , c=-1
 
D   =   b2 - 4ac  put the values in formula
      
D   =   (5)2 - 4(3)(-1)
D   =   25+12
D   =   37
D   =    positive but not perfect square that why  roots of this equation are real,unequal and irrational. 

3-    -x2+ 2x-1=0

solution
a= -1 , b= 2 , c= -1
 
D   =   b2 - 4ac  put the values in formula
      
D   =   (2)2 - 4(-1)(-1)
D   =   4-4
D   =   0
D   =  0 so the roots of this equation are real and equal. 

4-    x2+ 5x-6=0

solution
a= 1 , b= 5 , c= 6
 
D   =   b2 - 4ac  put the values in formula
      
D   =   (5)2 - 4(1)(6)
D   =   25-24
D   =   1
D   =  positive and perfect square so roots of this equation are real,unequal and rational. 



Posted by Unknown on 00:45  No comments »

Wednesday 11 October 2017

prove quadratic formula and steps to prove quadratic formula easily

Quadratic formula 

it is a formula which is to be used to solve quadratic equation easily.we proved this formula from standard form of quadratic equation. if we solve standard form of quadratic equation by using completing square method then we easily prove the quadratic formula. 

 lets prove. 


Posted by Unknown on 23:50  No comments »

define quadratic equation and methods to solve the quadratic equation

Define Quadratic equation.


An equation which have highest degree of variable x or y is square.in quadratic equation a,b and c are real numbers and a not equal to zero.

standard form of quadratic equation .

the standard form of quadratic equation is  ax2 + bx + c = 0
where a,b and c are coefficients or contents and x is known as variable(an unknown term)
it is a standard form of quadratic equation in x  variable but if we written the standard form of quadratic equation in y variable the it will be in this form ay2 + by + c = 0

Method to solve the quadratic equation.

there are three main and simple methods to solve the quadratic equation.

1- Factorization method.
2-Completing square method.
3- Method of quadratic formula.

examples of standard form of quadratic equation equation.

 1 -      3x2 + 10x + 7 = 0
 2 -      6x2 + 7x + 2 = 0
 3 -      7x2 _ 6x + 1 = 0
 4 -      a2x2 + abx - 2b2 = 0

Posted by Unknown on 03:02  No comments »

Wednesday 9 November 2016

Define Ratio,proportion and types of variation.

Define ratio?

                                Ratio is the relationship b/w two quantities.

Define proportion? 

                               Proportion is the Relationship b/w two Ratios.

TYPES OF PROPORTION

   There are two main types of proportion/variation.  
1) Direct proportion/ variation
2)Inverse proportion/variation 

Direct proportion/variation 

 A proportion in which one quantity is  increase/decrease and another quantity  are also  increase/decrease  then this type of proportion is know as direct proportion .
for example:
prize of 5 pens are RS 50 ,if you  have 100 rupees ,how many pens you purchase?
answer : we purchase 10 pens . 
In this question quantity of pens are increase because the rupees are also increase . 

Inverse proportion/variation 

A variation in which one quantity is increase but another is decrease them this type of variation is know as Inverse variation. 
for example:  5 masons can complete a building in 10 months ,10 masons can complete the same work in how many months?
answer:  5 months 
in this question the quantity of masons are increase but the months are decrease it is known as Inverse variation.
 
Posted by Unknown on 00:13  No comments »

Bookmark Us

Delicious Digg Facebook Favorites More Stumbleupon Twitter

Search

Follow Us